- Energy Self-shielding.
- Spatial Self-shielding.
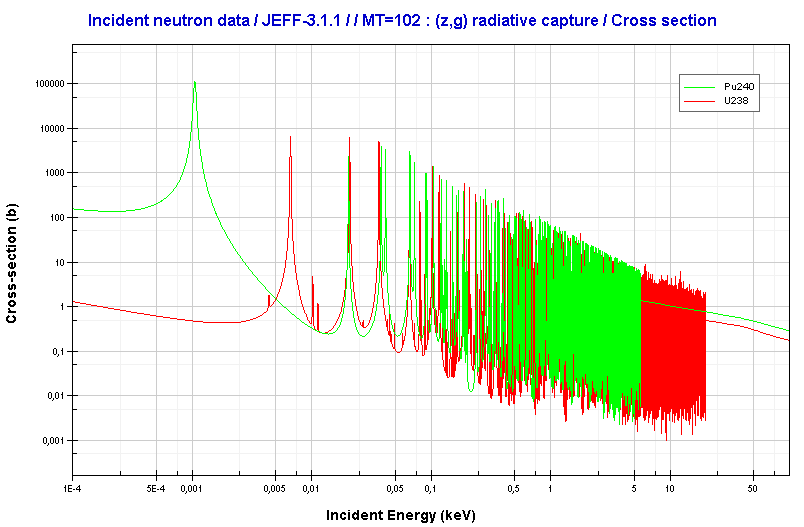
Energy Self-shielding
In each nuclear reactor, there is a characteristic neutron energy spectrum that, in the case of thermal reactors, also covers the resonance region of various materials (238U is important in this phenomenon). The vicinity of the resonance causes an increase in the neutron absorption probability when a neutron has energy near a resonance. This reduces the effective absorption per nucleus due to the depression of the energy-dependent flux Φ(E) near the resonance compared to a flat flux. At energies just below the resonance, where σa (E) becomes small again, the neutron flux reaches almost the same value above the resonance. This reduction in the energy-dependent neutron flux near the resonance energy is known as energy self-shielding.

Spatial Self-shielding
The basic fuel element of light water reactors is a fuel rod that contains fuel pellets made of uranium dioxide. For example, an 1100 MWe (3300 MWth) reactor core may contain 157 fuel assemblies composed of over 45,000 fuel rods and 15 million fuel pellets. The neutron moderator surrounds these fuel rods. The spatial self-shielding is a phenomenon primarily connected with this heterogeneity of the reactor core.
The fission neutrons are born in the fuel, but they are primarily moderated (slowed down) in the moderator. Thermal and epithermal neutrons return into the fuel to be absorbed and to cause further fission. With energy, self-shielding neutrons are more likely absorbed by the nuclei near the fuel surface. In this case, the surface layers of the fuel geometrically shield the inner layers from neutron flux, leading to a relatively lower neutron flux inside the fuel rod. For neutron energies near resonances (energy self-shielding), the depression of the energy-dependent flux is dramatic.
The spatial and energy self-shielding that occurs primarily in heterogeneous reactor cores causes a significant increase in the resonance escape probability (“p” from the four-factor formula) compared to homogeneous cores. Without the spatial self-shielding provided by the separation of fuel and moderator, values of keff = 1 are possible with natural uranium fuel only if heavy water is used as the moderator.
Another example:
In the nuclear industry, gadolinium is commonly used as a neutron absorber due to the very high neutron absorption cross-section of two isotopes 155Gd and 157Gd. In fact, their absorption cross-sections are the highest among all stable isotopes. 155Gd has 61 000 barns for thermal neutrons (for 0.025 eV neutron) and 157Gd has even 254 000 barns. Some power plants use gadolinium as a burnable absorber diluted in the fuel matrix in mixture Gd2O3 + UO2. Such a high absorption cross-section of gadolinium causes the fuel rod to burn up layer by layer (from the surface to inner layers). At the beginning of the cycle, this fuel rod behaves like being from neutron black material. At the beginning of the cycle, the higher content (i.e., from 4% to 6% of Gd2O3) of gadolinium causes a minimal decrease in the overall compensation of the core reactivity. Only the higher amount of such rods with burnable absorbers results in the overall decrease in core reactivity. This is also an example of a very strong effect of self-shielding.
Self-shielding and Doppler Broadening
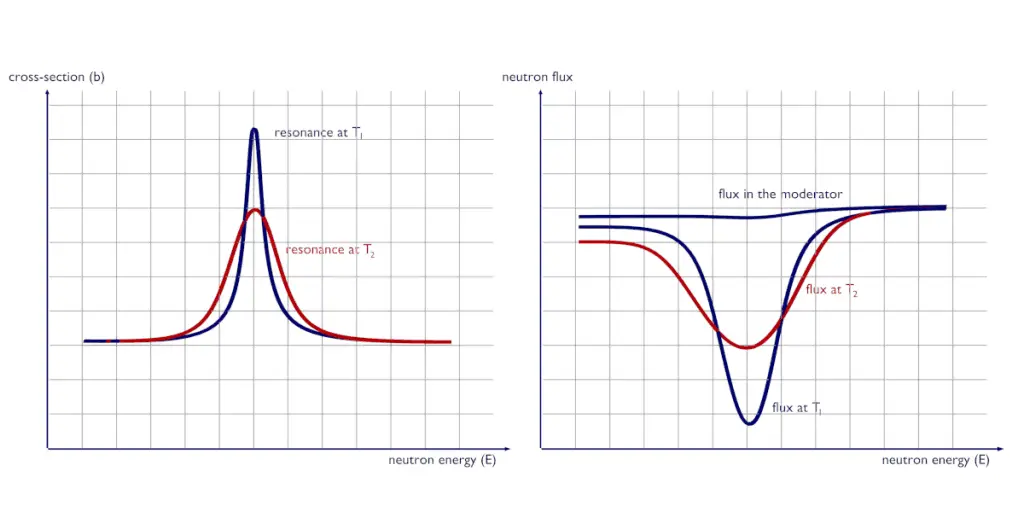
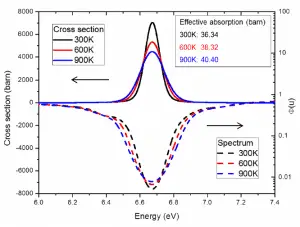
There is a further phenomenon connected with spatial and energy self-shielding. The energy self-shielding is significantly affected by the material temperature. It is closely connected with the Doppler broadening and the fuel temperature coefficient (also known as the Doppler coefficient). The material temperature affects the energy self-shielding through Doppler-broadening of the microscopic resonance cross-section. With an increase in fuel temperature, the thermal motion of target nuclei increases resulting in the broadening of spectral lines due to the Doppler effect. The wings of resonances are broadened while their peak magnitude decreases. The energy-dependent neutron flux closely follows the behavior of the changed resonances in a reverse manner, leading to a reduction in the self-shielding and an increase in the effective microscopic absorption cross-section. This, in turn, provides negative reactivity feedback against fuel temperature increase.